next up previous contents index
Next: The locally connected combs Up: Dendrites Previous: Simple examples

The locally connected fan

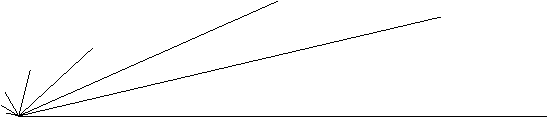
Another simple example is the locally connected fan F_\omega $. It is defined as the union of countably many straight line segments of length tending to 0, emanating from a point p$ and disjoint out of p$. Thus F_\omega $ is the dendrite with only one ramification point whose order is \omega$.

Figure 1.3.3: ( A ) locally connected fan F_\omega $
A.gif

The following mapping properties of F_\omega $ are known.

  1. F_\omega $ is universal in the class of all n$-ods for n \in \mathbb{N}$.
  2. Each open image of F_\omega $ is homeomorphic to F_\omega $, [Charatonik et al. 1990, Proposition 9.4, p. 42].
  3. A confluent mapping defined on F_\omega $ is open if and only if it is light, [Charatonik et al. 1990, Theorem 9.6, p. 42].

Here you can find source files of this example.

Here you can check the table of properties of individual continua.

Here you can read Notes or write to Notes ies of individual continua.
next up previous contents index
Next: The locally connected combs Up: Dendrites Previous: Simple examples
Janusz J. Charatonik, Pawel Krupski and Pavel Pyrih
2001-11-30