next up previous contents index
Next: Universal dendrites Up: Dendrites Previous: The locally connected fan

The locally connected combs

The next two examples are defined in [Arévalo et al. 2001, p. 3]. Let \overline {pq}$ stand for the straight line segment from p$ to q$ in the plane. In the plane, let a = (0,0), a_n = (1/n, 1/n), b_n = (1/n, 0)$ for each n \in \mathbb{N}$, and c = (-1,0)$. Define

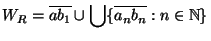
\displaystyle W_R = \overline{ab_1} \cup \bigcup \{\overline{a_nb_n}: n \in \mathbb{N}\}$   and\displaystyle \quad W = \overline{ca} \cup W_R.$

The dendrites W_R$ and W$ are called the comb W_R$ and the comb W$ , or (generally) locally connected combs .

See Figures A-B.

Figure 1.3.4: ( A ) comb W_R$
A.gif

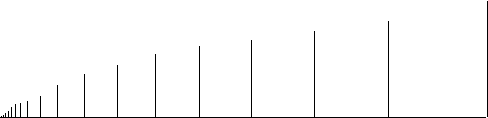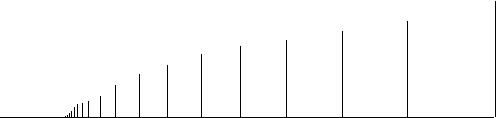
Figure 1.3.4: ( B ) comb W$
B.gif

The dendrites F_\omega $, W_R$ and W$ are exploited in the following characterizations.

  1. A dendrite is a tree if and only if it contains neither a copy of F_\omega $ nor of W_R$, [Arévalo et al. 2001, Theorem 3.1, p. 3].
  2. A dendrite has the set of all its end points closed if and only if it contains neither a copy of F_\omega $ nor of W$, [Arévalo et al. 2001, Corollary 5.4, p. 11].

Here you can find source files of this example.

Here you can check the table of properties of individual continua.

Here you can read Notes or write to Notes ies of individual continua.
next up previous contents index
Next: Universal dendrites Up: Dendrites Previous: The locally connected fan
Janusz J. Charatonik, Pawel Krupski and Pavel Pyrih
2001-11-30