




Next: Self-homeomorphic dendrites
Up: Dendrites
Previous: Other universal dendrites
In connection with the characterization of dendrites having
closed set of their end points (see
Property 2 in 1.3.4 above)
the classes of those dendrites that do not contain a copy of
 and those that do not contain a copy of the
comb
and those that do not contain a copy of the
comb  are
of some interest (see [Arévalo et al. 2001, p. 8]). The first
one is just the class of dendrites with finite orders of
ramification points, and it is known to have a universal
element according to Property 4
in 1.3.8. The other class has
also a universal element
are
of some interest (see [Arévalo et al. 2001, p. 8]). The first
one is just the class of dendrites with finite orders of
ramification points, and it is known to have a universal
element according to Property 4
in 1.3.8. The other class has
also a universal element  . Its construction, given in
[Arévalo et al. 2001, p. 9] is the following.
. Its construction, given in
[Arévalo et al. 2001, p. 9] is the following.
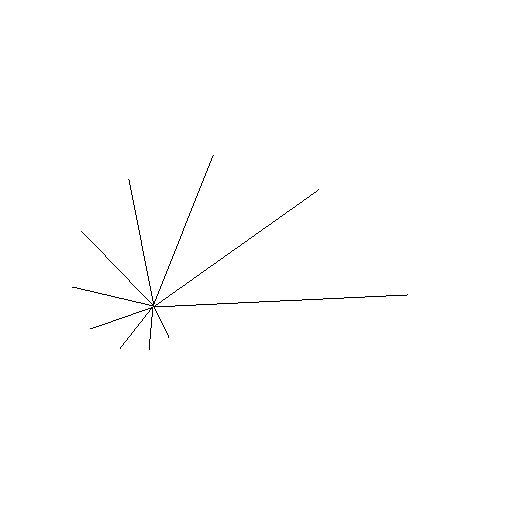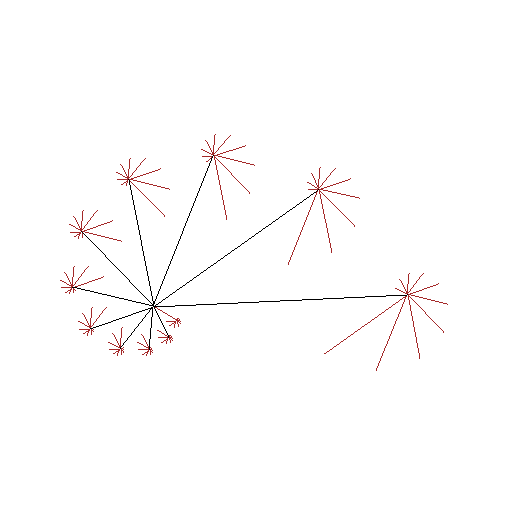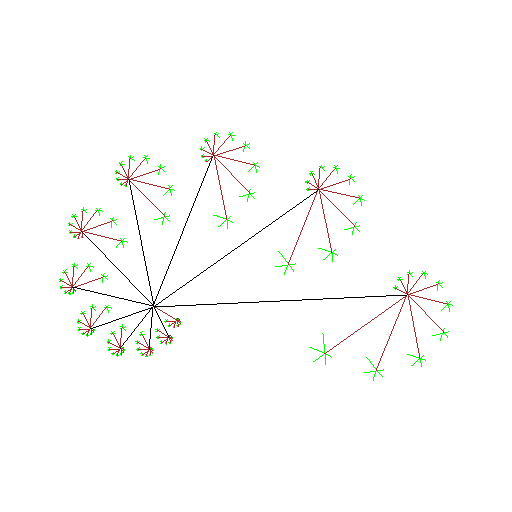
Let  be any point in the plane
be any point in the plane
 and let
and let
 be
a sequence of points in
be
a sequence of points in
 tending to
tending to  and such that no three
of the points
and such that no three
of the points
 are collinear. Define
are collinear. Define
 . Then
. Then  is homeomorphic to
is homeomorphic to
 . For every
. For every
 , let
, let  be a point in
be a point in
 such that for any fixed
such that for any fixed  the sequence
the sequence
 tends to
tends to  , no three of the points
, no three of the points
 are collinear, and that the continuum
are collinear, and that the continuum
 is a dendrite. We continue
constructing dendrites
is a dendrite. We continue
constructing dendrites
 in the same manner, such that we
get an increasing sequence of dendrites
in the same manner, such that we
get an increasing sequence of dendrites
 in
in
 having the property that the closure of their union is also a dendrite.
Finally define
having the property that the closure of their union is also a dendrite.
Finally define
The following properties of the
dendrite  are proved in [Arévalo et al. 2001, Theorems 4.5 and 4.6, p.
10].
are proved in [Arévalo et al. 2001, Theorems 4.5 and 4.6, p.
10].
- A dendrite
 is homeomorphic to the dendrite
is homeomorphic to the dendrite  if and only if
each of the following conditions is satisfied:
if and only if
each of the following conditions is satisfied:
 contains no copy of
contains no copy of  ;
;
- the set of all end points of
 is contained in the closure of
the set of all ramification points of
is contained in the closure of
the set of all ramification points of  ;
;
- all ramification points of
 are of order
are of order  .
.
- The dendrite
 is universal in the class
of all dendrites containing no copy of
is universal in the class
of all dendrites containing no copy of  .
.
See Figure A.
Figure 1.3.9:
( A ) dendrite 
 |
Figure:
( AA ) dendrite  - an animation
- an animation
 |
Here you can find source files
of this example.
Here you can check the table
of properties of individual continua.
Here you can read Notes
or
write to Notes
ies of individual continua.





Next: Self-homeomorphic dendrites
Up: Dendrites
Previous: Other universal dendrites
Janusz J. Charatonik, Pawel Krupski and Pavel Pyrih
2001-11-30
![]() be any point in the plane
be any point in the plane
![]() and let
and let
![]() be
a sequence of points in
be
a sequence of points in
![]() tending to
tending to ![]() and such that no three
of the points
and such that no three
of the points
![]() are collinear. Define
are collinear. Define
![]() . Then
. Then ![]() is homeomorphic to
is homeomorphic to
![]() . For every
. For every
![]() , let
, let ![]() be a point in
be a point in
![]() such that for any fixed
such that for any fixed ![]() the sequence
the sequence
![]() tends to
tends to ![]() , no three of the points
, no three of the points
![]() are collinear, and that the continuum
are collinear, and that the continuum
![]() is a dendrite. We continue
constructing dendrites
is a dendrite. We continue
constructing dendrites
![]() in the same manner, such that we
get an increasing sequence of dendrites
in the same manner, such that we
get an increasing sequence of dendrites
![]() in
in
![]() having the property that the closure of their union is also a dendrite.
Finally define
having the property that the closure of their union is also a dendrite.
Finally define
![]() are proved in [Arévalo et al. 2001, Theorems 4.5 and 4.6, p.
10].
are proved in [Arévalo et al. 2001, Theorems 4.5 and 4.6, p.
10].