Pseudo-arc

A. Lelek, ZBIORY (Sets), PZWS Warszawa 1966, pages 90-95.

The first hereditarily indecomposable continuum has been constructed by B. Knaster in 1922. This continuum K, discovered by Knaster, is just named the pseudo-arc or Knaster's continuum. The pseudo-arc K is obtained as the common part of a decreasing sequence of continua in the plane, every of which is the union of finitely many discs, forming a chain of sets.

We will consider chains

$$\mathcal D = (D_1, D_2, \dots, D_k)$$

of discs D_i in the plane, having the property that any two consecutive disc D_i and D_i+1 have interior points in common, i.e., that their intersection -- nonempty by assumption -- is not contained in the boundary of any of them (Figure A). Discs D_i are called links of the chain $\mathcal D$. The union of all links of the chain $\mathcal D$ is a continuum. Observe that if we delete from the chain $\mathcal D$ a link which is not an end link, i.e., a link D_i such that 1 < i < k, then the union of the remaining links is a not connected set, having two components.

Let two chains of discs $\mathcal D$ and $\mathcal D'$ be given. We say that the chain $\mathcal D'$ refines the chain $\mathcal D$ if each link D'_s of the chain $\mathcal D'$ is contained in the interior of at least one link D_i of the chain $\mathcal D$, i.e., if for every $D'_s \in \mathcal D'$ there exists a link $D_i \in \mathcal D$ such that $D'_s \subset D_i$ and that the disc D'_s has no common points with the boundary of the disc D_i (Figure B).

We say that the chain $\mathcal D'$ is crooked in the chain $\mathcal D$ if it refines $\mathcal D$ and if, for every pair of links D_i and D_j of the chain $\mathcal D$ such that

i + 2 < j, (1)

and for every pair of links D'_s and D'_v of the chain $\mathcal D'$, intersecting the links D_i and D_j respectively, i.e.,

$$D_i \cap D'_s \ne \emptyset \ne D_j \cap D'_v,$$

there are, in the chain $\mathcal D'$, links D'_t and D'_u, lying between the link D'_s and D'_v in the same order, i.e.,

$$s < t < u < v \quad \mbox { or } \quad s > t > u > v,$$

such that

$$D'_t \subset D_{j-1} \quad \mbox { and } \quad D'_u \subset D_{i+1}.$$ (2)

In other words, a part of the chain $\mathcal D'$ which is contained between the links D'_s and D'_v has to form a fold in the chain $\mathcal D$ between the links D_i and D_j (Figure C). The chain $\mathcal D'$, to go from the link D_i to the link D_j has first to come to the link D_j-1, next go back to the link D_i+1, and only after this it can reach the link D_j. Such a fold must exist for every two links D_i and D_j having no adjoining (i.e. neighbor) link in common.

Now, let there be given in the plane two points a and b, and an infinite sequence

$$\mathcal D_1, \mathcal D_2, \dots , \mathcal D_n, \dots$$

of chains of discs, satisfying, for every $n \in \{1, 2, \dots \}$, the following conditions:

   1^o

the point a belongs to the first, and the point b to the last link of the chain $\mathcal D_n$;

   2^o

the diameter of every disc in the chain $\mathcal D_n$ is less than $\frac 1n$;

   3^o

the chain $\mathcal D_{n+1}$ is crooked in the chain $\mathcal D_n$.

Thus, for example, the chain $\mathcal D_2$ is crooked in the chain $\mathcal D_1$, and the chain $\mathcal D_3$ is crooked in the chain $\mathcal D_2$ (on Figure D only a part of the chain $\mathcal D_3$ is presented). The foldings are more and more condensed and they overlapped themselves.

Denote by K_n the union of all links of the chain D_n. From condition 3^o it follows in particular that the each next chain refines the previous one, and therefore the continua K_n form a decreasing sequence

$$K_1 \supset K_2 \supset \dots \supset K_n \supset \dots$$

The Pseudo-arc K is defined as the common part of all continua K_n, i.e.,

$$K = \bigcap \{K_n: n \in \Bbb N\}.$$

By virtue of condition 1^o points a and b belong to the continuum K, thus it is nondegenerate. We shall prove that the continuum K is hereditarily indecomposable.

Let $K' \subset K$ be an arbitrary continuum. To prove that the continuum K is indecomposable it is enough to show that every continuum $Y \subset K'$ distinct from K' is nowhere dense in K'. Consider an arbitrary point $p \in Y$ and number $\varepsilon > 0$.

Since $K' \ne Y$, hence there is a point $q \in K' \setminus Y$ which therefore is at a distance at least $\eta > 0$ from each point $y \in Y$, where $\eta$ is a fixed number, independent from y. In fact, if not, then some points of the set would be arbitrarily close to the point q, and so the point q would belong to the closure of the set Y, which is impossible, because the set Y is closed, and it does not contain the point q. Let us take a natural number n so large that the inequalities are satisfied

$$\frac 2n < \varepsilon \quad \mbox { and } \quad \frac 3n < \eta.$$

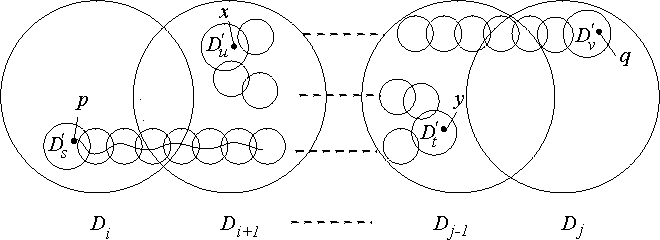
Denote by D_i and D_j (with $i \le j$) the links of the chain $\mathcal D_n$ whose union contains the points p and q. Since $p \in Y$, hence $\rho (p,q) \ge \eta$, (here $\rho$ means the metric in the plane) and thus it follows that between the links D_i and D_j there are at least two links of the chain $\mathcal D_n$. Indeed, in the opposite case the links D_i and D_j would have a neighbor common link, and choosing suitable points p' and q' in the intersections of this link with the links D_i and D_j we would have

$$\rho (p,q) \le \rho (p,p') + \rho (p',q') + \rho (q',q) < \frac 1n + \frac 1n + \frac 1n = \frac 3n < \eta$$

by condition 2^o. Thus we can assume that the indices i and j satisfy the inequality (1). Further, we can assume also that the point p belongs to one, and the point q to the other of the links D_i and D_j.

Denote by D'_s and D'_v the links of the chain $\mathcal D_{n+1}$ which contain points p and q, respectively. Additionally we assume that $p \in D_i$. In the opposite case, i.e. if $p \in D_j$, the further part of the proof runs in the same way (with changing of the roles of the links D_i and D_j as well as D_i+1 and D_j-1).

So,

$$p \in D_i \cap D'_s \quad \mbox { and } \quad q \in Dj \cap D'_v,$$

whence we infer, by virtue of condition 3^o and the definition of folding of chains, that there are two links D'_t and D'_u in the chain $\mathcal D_{n+1}$ which lie between the links D'_s and D'_v in the same order and which satisfy the inclusions (2). The union of the links of the chain $\mathcal D_{n+1}$ distinct from the link D'_u is a not connected set (see above) containing the point p in one component, and the point q in the other. Since the continuum K' joins the points p and q and is contained in the union K_n+1 of all links of the chain $\mathcal D_{n+1}$, hence K' must pass thru the link D'_u. Thus there is a point

$$x \in K' \cap D'_u$$

(Figure E). We shall prove that the point x does not belong to the continuum Y. Indeed, if it would be $x \in Y$, then by the same reason as previously for the continuum K' the continuum Y would have to pass thru the link D'_t. Then there would exist a point

$$y \in Y \cap D'_t,$$

which would be in the link D_j-1 according to (2). But the neighbor links D_j and D_j-1 have their diameters less than $\frac 1n$ by condition 2^o, and therefore we would have

$$\rho (y,q) < \frac 2n < \frac 3n < \eta,$$

which is impossible because $y \in Y$.

So, the point x belongs to the set $K' \setminus Y$ and to the link D_i+1 simultaneously. The neighbor links D_i and D_i+1 have diameters less than $\frac 1n$, whence it follows that

$$\rho (p,x) < \frac 2n < \varepsilon .$$

Since $\varepsilon$ was an arbitrary positive number, hence, in this way we have proved in that there are points of the set $K' \setminus Y$ which lie arbitrarily closely to the point p. The point p was an arbitrary point of the continuum Y. Thus we have proved that the continuum Y is nowhere dense in K', and the proof of hereditary indecomposability of the continuum K is finished.

An example of a chain crooked in a chain with 7 links is on Figure F.

Figure ( A ) a chain with links, construction of the Pseudo-arc


Figure ( B ) refining a chain, construction of the Pseudo-arc


Figure ( C ) crookedness used in the construction of the Pseudo-arc


Figure ( D ) crookedness used in the construction of the Pseudo-arc


Figure ( E ) crookedness used in the construction of the Pseudo-arc


Figure ( F ) an example of a chain crooked in a chain with 7 liks, construction of the Pseudo-arc


Source files: a.cdr . a.eps . a.gif . a.txt . b.cdr . b.eps . b.gif . b.txt . c.cdr . c.eps . c.gif . c.txt . d.cdr . d.eps . d.gif . d.txt . e.cdr . e.eps . e.gif . e.txt . f.cdr . f.eps . f.gif . f.txt . latex.tex . title.txt .

Here you can read Notes or write to Notes.