universal

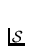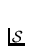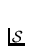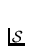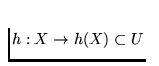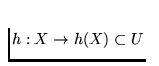Let a class $\mathcal S$ of spaces be given. A member U of $\mathcal S$ is said to be universal for $\mathcal S$ if every member of $\mathcal S$ can be embedded in U, i.e., if for every $X \in \mathcal S$ there exists a homeomorphism $h: X \to h(X) \subset U$. Accordingly, a dendrite is said to be universal if it contains a homeomorphic image of any other dendrite.