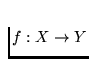 be a mapping between continua. Then the
induced mapping
be a mapping between continua. Then the
induced mapping
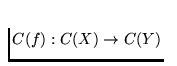 is defined by
C(f)(A) =
f(A), where A in the left member of the equality means an element of
C(X), while in the right one it is understood as a subcontinuum of X
(see [64, (0.49), p. 23]).
is defined by
C(f)(A) =
f(A), where A in the left member of the equality means an element of
C(X), while in the right one it is understood as a subcontinuum of X
(see [64, (0.49), p. 23]).